(The position is now filled).
We have perfected methods to detect cold atom clouds released from a trap in three dimensions and with single atom sensitivity. We have been using this method to perform fundamental experiments on correlations and entanglement produced by non-linear processes in Bose-Einstein condensates. An example is our observation of the Hong-Ou-Mandel effect [1].
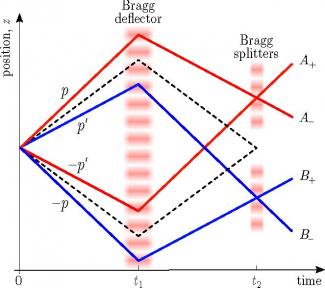
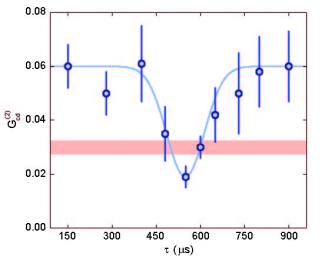
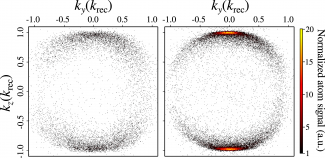
The group has recently developed a two-particle interferometer in momentum space [2], see figure. Starting from a two-particle entangled state, |p,-p〉 + |p',-p'〉, this configuration can test a Bell inequality. This would be the first time that such inequalities have been tested for the motional degrees of freedom of freely falling, massive particles.
To perform a Bell test in this configuration, it is necessary to have interferometric phase control (𝜑A and 𝜑B ). A first part of the internship will be to implement the phase control and check it on a standard one-particle interferometer. Preliminary experiments are underway to optimize the stability and speed to produce the expected entangled state; the intern will participate in it. He/she will then participate in the Bell experiment i.e. acquisition of the data and its analysis.
We use a non-linear process in a Bose-Einstein condensate to produce entangled atom pairs reminiscent of four-wave mixing in optics. The condensate is loaded in a moving optical lattice. The band structure of the lattice modifies the dispersion relations of the atoms so that correlated atom pairs are produced spontaneously. This process is slightly multi-mode and therefore the resulting state is multi-particle entangled. An extension of the proposed scheme to look at entanglement on a larger set of modes is also envisioned.
Bibliography references
[1] R. Lopes et al., Nature 520, 66 (2015),
[2] P. Dussarrat et al., Phys. Rev. Lett. 119, 173202 (2017), arXiv:1707.01279.
[3] Perrier et al. SciPost 7, 002 (2019)
Do not hesitate to get in touch with Chris or Denis
- christoph[dot]westbrook[at]institutoptique[dot]fr
- denis[dot]boiron[at]institutoptique[dot]fr